Online height transformation in the border areas of Germany, Austria and Switzerland (D-A-CH height transformation) — Expert mode

Explanations

Background on height systems

Heights and zero-level surfaces in Germany, Austria and Switzerland differ for historical and geophysical reasons.

What we experience as “heights” in daily life (physical heights, “heights above sea level”) depends on the Earth’s gravity field. The concept of the geoid, i.e., the hypothetical surface of an undisturbed ocean extended at land, is understood as the idealized reference surface for heights.

Consequently, models of (quasi)geoid heights are used as height reference surface for GNSS-based height determination, i.e., for the transformation of observed ellipsoidal heights into physical heights (see Fig. 1). In the generalized formula

h = H + U

h is the ellipsoidal height, H denotes the respective physical height, and U represents the undulation value of the corresponding height reference surface (i.e., geoid height N or quasigeoid height ζ).
 

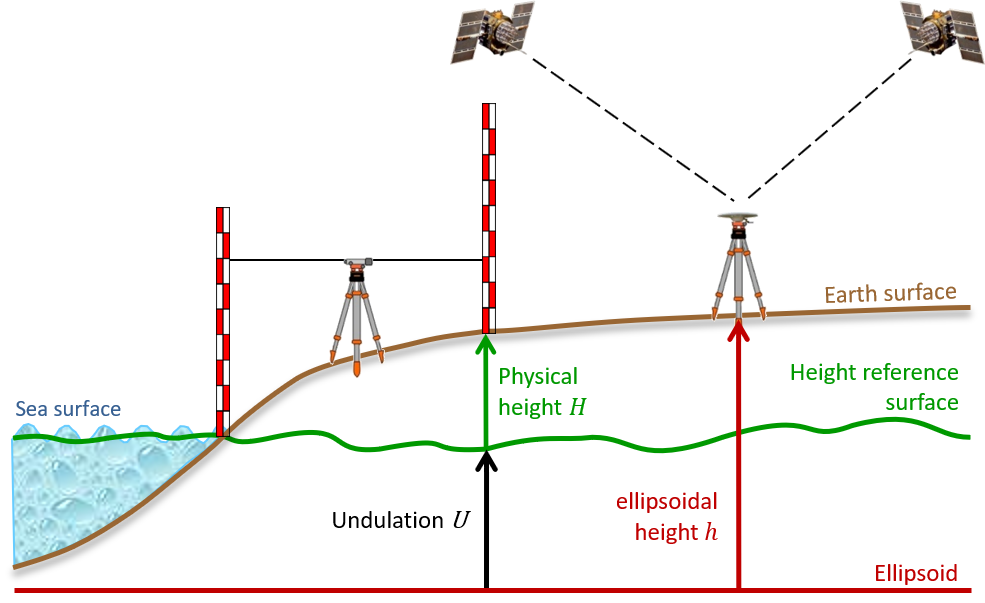
Fig. 1 Schematic view of heights and height reference surfaces

Fig. 1 Schematic view of heights and height reference surfaces
 

Unfortunately, “the” mean sea level (and, thus, “the” geoid) does not exist. The real oceans underly ocean dynamics which are driven by the hydro-mechanic settings of the coast as well as oceanographic and atmospheric parameters. Therefore, even the long-term mean sea level is not an equilibrium surface. Thus, the observed mean sea level at a specific cite does not coincide with the global mean sea level resp. geoid. The zero level of a regional or national height network and the according geoid model is always subject to a datum definition and depends on the location and epoch of the mareograph site which is taken as a reference.

Like all classical (optical) geodetic measurement techniques, height measurements need to be corrected for the influence of the local gravity field. The adjustment of national and continental height networks is done by means of so-called geopotential numbers C or potential differences ΔW = g ⋅ ΔH between two points (i.e., in units of the potential energy of a unit mass, which means “acceleration multiplied by distance” instead of “force multiplied by distance”). In order to convert these potential numbers into metric heights, they must be divided (normalized) by a gravity value. Different types of heights are defined, depending on which kind of gravity value is used for the correction and/or the normalization. Today, normal heights (e.g., Germany, system DHHN2016) and orthometric heights (e.g., Switzerland, new system LHN95) are the most commonly used. The choice of the height type for the height networks of the individual countries has theoretical, practical and historical reasons, which shall not be discussed here. A comprehensive overview on height types can be found in Schlatter and Marti (2007, in particular sections 2.3, 2.6 and Fig. 2.6-1, in German), whereas a short summary has been compiled in Tab. 1.
 

Tab. 1 Fundamental properties and definitions of height types and height reference surfaces. See Fig. 2 for a visualization of the respective point levels used in the formula items.

Attribute Uncorrected heights Normal-orthometric heights HNO Normal heights HN Orthometric heights HO
Height reference surface Implicit Implicit Quasigeoid Geoid
Undulation Implicit Implicit Height anomaly
(quasigeoid height) ζ		 Geoid height N
Gravity corrections to the raw leveled heights None Spheroidal only using normal gravity Free-air anomalies using actual gravity Free-air anomalies using actual gravity
Theoretical loop-closure error? Yes Yes No No
Based on true geopotential numbers C? No No Yes Yes
Gravity value GH to be used for scaling of geopotential number to metric height value
H = C/GH		 Not applicable Not applicable Mean normal gravity along plumbline between ellipsoid and telluroid γ(Q0,Q) Mean actual gravity (approximanted) along plumbline between geoid and the Earth's surface g(P0,P)
Definition of the disturbing potential T (actual minus normal potential) for the height reference surface Not applicable Not applicable On the Earth's surface TP On the geoid T(P0)
Gravity vale GU to be used for scaling of disturbing potential to metric undulation value
U = T/GU		 Not applicable Not applicable Normal gravity on the telluroid γQ (or on the Earth's surface γP without loss of accuracy) Normal gravity on the ellipsoid γ0
Free of density hypothesis? Yes Yes Yes No

 

Fig. 2 Visualization of the respective reference levels in the formulas for heights and undulations as used in Tab. 1

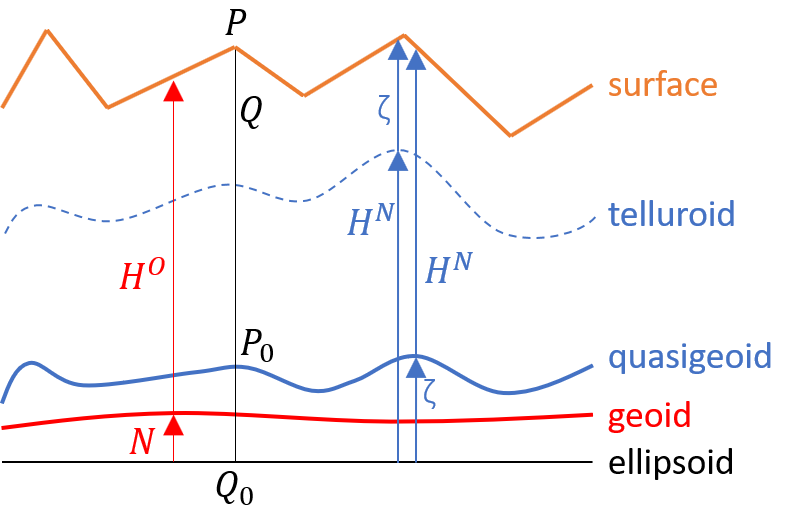
Fig. 2 Visualization of the respective reference levels in the formulas for heights and undulations as used in Tab. 1
 

Although orthometric heights and normal heights are routinely computed and provided for scientific applications in Austria and Switzerland, the official heights systems of these countries based on normal-orthometric heights (Austria, System MGI) or even uncorrected heights (Switzerland, “old” System LN02) remain to be in use.

Different height types are the prevailing reason why height differences between countries vary along the course of national borders. For example, height numbers in the Swiss system LN02 are approx. 32 cm larger than in the German system DHHN2016 close to Basel, whereas near Schaffhausen the difference is approx. 36 cm.
 

The D-A-CH height transformation service

The application allows the transformation between the supported national height system realizations (physical heights) and the national ETRS89 realizations (ellipsoidal heights). Transformation paths are defined following a two-step approach as shown in Fig. 3. First, if necessary, the height is transformed into an EVRS-compatible normal height by means of the official national (quasi)geoid models or height transformation models. Then the inverse transformation into the target system is performed. If possible, an alternative transformation path (e.g. via ellipsoidal heights) is used in order to compute and output a DELTA value as accuracy indicator (analogously to a “loop closure error” of the models in the transformation scheme).

Transformation grids between the national height system realizations and the current realizations of the EVRS (EVRF2019) have been derived by the UELN Computing Centre (M. Sacher, c/o BKG). Along the national borders these typically show only a small variation in the order some millimeters. On the other hand, national height reference surfaces and height transformation grids usually have declared accuracies of 1-3 cm, which was tested and confirmed for sample points along the borders. Consequently, in the “DACH transformation service”

Since the national height grids are limited to the respective national territories including small buffer zones, the cross-border transformation operations (such as, e.g., DHHN2016 to MGI) have been limited to a 4 km zone on both sides of the border. Conversions from physical heights to ellipsoidal heights within a country (e.g. DHHN2016 to ETRS89/DREF91 Realization 2016) are possible without restrictions though.
 

Geodetic reference frames in the D-A-CH countries

Tab. 2 Overview and relevant specifications of height reference frames in the D-A-CH countries. Transformation grids have to be added to obtain heights in the reference frame in the subsequent row (unless otherwise stated). For reasons of clarity, unofficial versions in terms of normal heights of Austria and Switzerland (see Fig. 3) are not included here.

Reference frame Height type Datum points Tide gauge/
Zero level		 Reference epoch Transformation grid Remarks
Germany
DHHN2016
(“H. ü. NHN”)		 Normal height 72 points NAP (1684) 2004-2013
(mostly 2006-2012)		 GCG2016 quasigeoid
ETRS89/DREF91 Realization 2016 ellipsoidal 2008.46 Heights consistent with ETRF2000
Austria
MGI
(„H. ü. A.”)		 normal-orthometric Hutbigl Triest (1875) 1950-2011 Höhen-Grid
AT_NAP_OH orthometric Hutbigl NAP (1684) 1950-2011 Austrian Geoid 2008
ETRS89/ETRF2000 Austria 2002.45 ellipsoidal 2002.45
Switzerland
LN02 uncorrected Répère du Niton (RPN) Marseille forced to nodal points of network 1861-1891 CHLN02
(to CHTRF20xx)
LHN95 orthometric Zimmerwald Marseille 1993.0 CHGeo2004 geoid LN02 height of RPN preserved
CHTRF20xx ellipsoidal 1993.0 Height offset to ETRF2000

 

<i>Fig. 3</i> Simplified transformation scheme of the D-A-CH height transformation service (i.e., assuming a constant level difference between the national normal heights and EVRS, ellipsoidal heights and ETRF2000 accordingly)

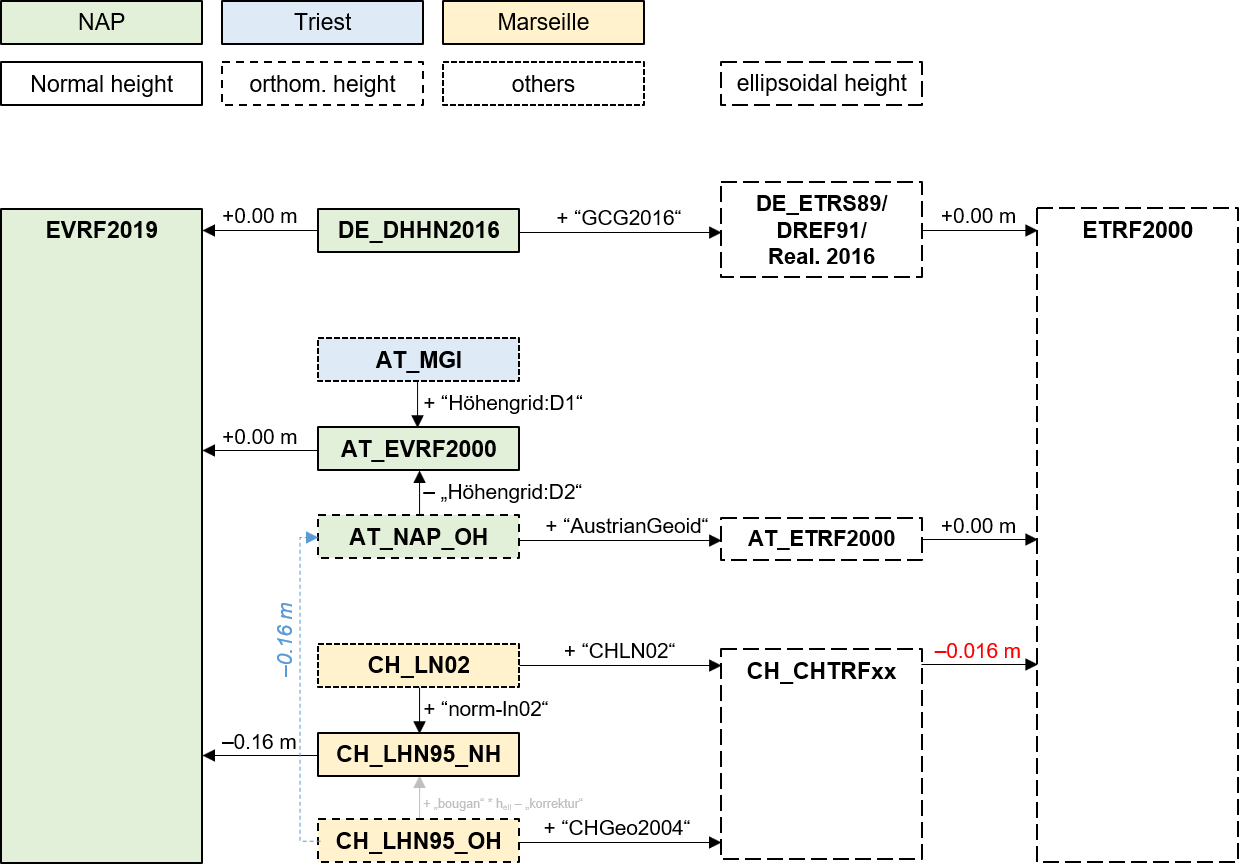
Fig. 3 Simplified transformation scheme of the D-A-CH height transformation service (i.e., assuming a constant level difference between the national normal heights and EVRS, ellipsoidal heights and ETRF2000 accordingly)
 

A note on “Normalnull (NN)” and “Normalhöhennull (NHN)” in Germany

For historical reasons, a number of different height systems (and height system realizations) had been used in Germany, too. Here, in the D-A-CH height transformation application, these German legacy systems are deliberately left out. A comprehensive overview can be found in Sacher and Liebsch (2018, Tab. 2.2, p. 23).

The term Normalnull (NN), which was used for the old German height systems before 1992 (except the GDR), does not refer to the application of normal heights (in fact, these heights were normal-orthometric!) but to the definition of the zero level, the Normal Amsterdam’s Peil (NAP).

In contrast, the zero level surface of the normal heights since 1992 (DHHN92, DHHN2016) is refered to as Normalhöhennull (NHN). It is realized by quasigeoid models (DHHN92: GCG05/GCG2011, DHHN2016: GCG2016).
 

Further information / Literature

DE – Germany

AT - Austria

CH – Switzerland

 

back

Imprint

Privacy

to top